
By Nic Lewis reposted from Climate Etc.
James Hansen’s latest paper “Global warming in the pipeline” (Hansen et al. (2023)) has already been heavily criticized in a lengthy comment by Michael Mann, author of the original IPCC ‘hockey stick’. However Mann does not deal with Hansen’s surprisingly high (4.8°C) new estimate of equilibrium climate sensitivity (ECS)1ECS is defined as the global mean surface warming for a doubled CO2 concentration after so-called fast-feedbacks have been fully activated and the ocean has reached equilibrium (which it approaches within a thousand or so years). Earth system sensitivity (ESS), which also includes very slow feedbacks such as those from changes in ice sheets, represents global warming arising over much longer timescales. ESS is relevant when studying paleoclimate changes, but not when projecting changes over the next few centuries.. This ECS estimate is 60% above Hansen’s longstanding2E.g., in Hansen et al (2013) Climate sensitivity, sea level and atmospheric carbon dioxide. Phil Trans Roy Soc A. https://doi.org/10.1098/rsta.2012.0294 previous estimate of 3°C. It is Hansen’s new, very high ECS estimate drives, in conjunction with various questionable subsidiary assumptions, his paper’s dire predictions of high global warming and its more extreme concluding policy recommendations, such as ‘solar radiation management’ geoengineering.
Hansen’s new 4.8°C ECS estimate is well above the best estimate of 3°C reached in the IPCC’s latest scientific assessment report (AR6), lies outside the AR6 likely (66%) range of 2.5–4°C and is almost at the top of the AR6 90% uncertainty range of 2–5°C.3Some of the latest generation (CMIP6) global climate models do have ECS values above 5°C, but they are generally regarded by climate scientists as being too sensitive.
Both Hansen’s new ECS estimate and his earlier estimate are based primarily on information about paleoclimate changes, particularly the extensively studied transition from the last glacial maximum (LGM) some 20,000 years ago to the preindustrial Holocene. But is his new estimate (or indeed his earlier estimate) justified by the evidence?
Hansen’s primary LGM to Holocene based ECS estimation.
For his LGM-based ECS estimate, Hansen assumes a 7.0°C rise in global mean surface temperature (GMST) between the LGM and preindustrial Holocene. This value is 56% above the 4.5°C rise that Hansen used previously2. Hansen cites three studies in support of his 7.0°C LGM cooling estimate (which comes from the second of these): Tierney et al. (2020), Osman et al. (2021) and Seltzer et al. (2021).
I published in 2021 an article that was heavily critical of the Osman et al. data-assimilation (reanalysis) based temperature reconstruction. Their reconstruction uses only ocean sea surface temperature (SST) proxies and is based on a single climate model that simulates an unusually cold LGM state4Actually two slightly different versions, iCESM1.2 and iCESM1.3, of the same underlying CESM1 GCM. and, strongly influenced by the model simulations, produced a 7°C estimate of LGM cooling.5Supporting my critique of their reanalysis, I showed that its co-located estimates were uncorrelated with values from the cave speleothem proxies it used. Moreover, unlike proxy-based reconstructions (e.g., Caufman et al. (2020)), which show early Holocene GMST 5,000–9,000 years ago being circa 0.5°C higher than late preindustrial (1750) GMST, their reanalysis showed that period as being significant cooler than late preindustrial GMST. The proxy-only based reconstruction in the submitted version6The final, published version of Osman et al. was slightly different, with no mention of the change in the peer review files, and cooled marginally more near and at the LGM. of Osman et al. appeared more reasonable, and as shown in my article implied LGM to preindustrial GMST change of about 4.5°C.7Using a more recent model generation than the older ones Osman et al. used to derive the ratio used to convert their ex-polar sea surface temperature (SST) proxy-based estimates to GMST.
The Tierney et al. estimate of LGM cooling (6.1°C)8No explanation is available of why, disconcertingly, this differs from the 5.9°C in their submitted manuscript. is based on a much larger set of SST proxies, which includes all those employed by Osman et al. used, but similarly uses no land temperature proxies. Tierney et al. uses the same single-model data-assimilation temperature reconstruction method as does Osman et al.9The Osman et al. cooling estimate may be greater because they use only a subset of Tierney et al.’s proxies, and have no proxy coverage at all in the central Pacific ocean. Therefore, my criticisms of Osman et al.’s reanalysis-derived LGM cooling estimate very largely apply also to Tierney et al.’s estimate.
The Seltzer et al. 5.8 ± 0.6 °C LGM cooling estimate is for land only and is limited to 45°S–35°N, so does not represent GMST cooling. The authors use a groundwater-based proxy type and a complex model to convert proxy values to surface temperatures. I have concerns about some aspects of their methods.10While Seltzer et al. validated their model on modern proxy data, it may not be valid for conditions at the LGM, when factors such as vegetation cover, rainfall patterns, and seasonal temperature and precipitation cycles were different. An additional concern is that Seltzer use two alternative methods to select LGM samples, which give noticeably different LGM cooling estimates (5.8°C and 4.8°C). They prefer the method giving the higher estimate, however it will tend to pick out particularly cold temperatures from datasets, and so may overestimate LGM cooling. Interestingly, the average of the Annan et al. LGM reconstruction’s cooling estimates for grid cells co-located with the 15 proxies that Seltzer et al. use is close to the 4.8°C estimate that Seltzer et al. obtain using their second method. Moreover, their 5.8°C land cooling estimate is based on the error-weighted average of only 15 groundwater records, which show widely varying cooling11Thus, excluding two particularly cool, low assessed uncertainty proxy estimates would reduce the estimate by 0.4°C. and may not be adequately representative of 45°S–35°N land areas. Seltzer et al. completely ignore the uncertainty associated with these issues. Their estimate is inconsistent with most proxy-derived estimates of mean ocean cooling over 45°S–35°N, which casts further doubt on its reliability.
I regard the recent Annan et al. (2022) LGM temperature reconstruction as producing a more reliable estimate of GMST cooling than the Tierney et al. (2020) and Osman et al. (2021) studies that Hansen et al. relies upon. The Annan et al. reconstruction uses a similar data-assimilation method to those studies, but with several key differences. Unlike them it uses a simulations from large set of acceptably dissimilar climate models, rather than a single model. Importantly, unlike those two studies, Annan et al. scale the model simulated temperature changes so that the initial guess model cooling estimates used are approximately centered on the data, to minimize model-generated bias. Moreover, Annan et al. use land temperature proxies as well as a larger set of ocean SST proxies to adjust the initial guess. They use the same SST proxy dataset as Tierney et al., but extend its coverage with data from a widely used earlier SST dataset, which in particular reduces Tierney et al.’s huge gaps in coverage of the Pacific ocean.
As a result of the much better proxy coverage, the use of multiple models and the debiasing step, the 4.5°C estimate of GMST cooling at the LGM that the Annan et al. reconstruction produces should be much more realistic than the Tierney et al. (2020) and Osman et al. (2021) estimates that Hansen uses. Indeed, Annan et al. criticize Tierney et al.’s approach, using very cold simulations from a single model, pointing out that it will produce a cool reconstruction regardless of whether the data points to a milder LGM climate.
By coincidence12Lewis (2023) was submitted earlier than was Annan et al (2022)., Annan et al.’s 4.5°C GMST LGM cooling estimate is the same value as I adopted for the LGM derived climate sensitivity estimation in Lewis (2023)13Lewis, N., 2023. Objectively combining climate sensitivity evidence. Climate Dynamics, 60(9), pp.3139-3165.. That study reworked Sherwood et al. (2020), a very influential World Climate Research Programme linked review of climate sensitivity evidence, which had assessed the LGM to be 5°C cooler than preindustrial.
Hansen’s ECS estimate is increased not only by adoption of an unrealistically large LGM cooling value, but also by very low estimates14Hansen’s estimate of the greenhouse gas forcing change, relative to that from a doubling of CO2 concentration, is 27% lower than the value assessed by Sherwood et al. and also adopted in Lewis (2023). Moreover, Hansen ignores the significant dust aerosol forcing change assessed by Sherwood et al., likewise also adopted in Lewis (2023). for the various changes in forcing between the LGM and the preindustrial Holocene15In the formula for estimating ECS, the change in forcing (ΔF) forms the denominator: ECS = F2×CO2 × ΔT/ΔF, where ΔT is the GMST change in equilibrium and F2×CO2 is the forcing from a doubling of atmospheric CO2 concentration. All forcing changes given in this article are for effective radiative forcing (ERF), the principal forcing metric used in AR6.. Hansen’s central estimate for the total Holocene to LGM forcing difference is −5.75 W/m2, much smaller than the −8.63 W/m2 assessed by Sherwood et al.(2020) and even further below the −8.93 W/m2 best estimate adopted in Lewis (2023). The LGM section of Table 1 in the Appendix gives details of all the data-variable estimates used to form the Sherwood et al. (2020), Lewis (2023) and Hansen et al. (2023) LGM–preindustrial Holocene based ECS estimates.
The combination of the large GMST change and small forcing change that Hansen adopts for the LGM results in a central ECS estimate of 4.8°C. By comparison, the Lewis (2023) LGM-based ECS estimate was 2.2°C, somewhat less than the corresponding ECS estimate from Sherwood et al. of 2.8°C.16Sherwood et al. (2020) and Lewis (2023) focused on a climate sensitivity measure, S, an easier to estimate approximation to ECS that is normally derived for global climate models instead of ECS. They accordingly converted their underlying paleoclimate ECS estimates into estimates of S. Both these estimates are well below the lower bound of the 3.6 – 6.0 °C ECS 95% uncertainty range for ECS that Hansen estimates.
Hansen supports his LGM-based ECS estimate with one based on the difference in GMST between the LGM and the previous, Eemian, interglacial period. His estimate is therefore inflated by his assumption that the LGM was very cool. Moreover, compared to the LGM there is even greater uncertainty in Eemian GMST, ice-sheet forcing, and other non-CO2 forcing. IPCC AR6 mentions all these drawbacks and additionally points outs that accounting for varying orbital forcing is challenging for this period. Sherwood et al. (2020) did not attempt to estimate climate sensitivity from the Eemian to LGM or any other pre-LGM climate transition because the data available are far more limited than for the much more extensively studied LGM. I likewise did not do so in Lewis (2023), nor do I attempt such an estimate here.
Hansen’s analysis of the PETM event and its implications.
Hansen also considers the Paleocene-Eocene Thermal Maximum (PETM) warming event some 56 million years ago, adopting a best estimate for the warming involved of 5.6°C and assuming, as did Sherwood et al. (2020) and Lewis (2023), that greenhouse gases alone produced the increase in forcing that caused the warming.
Rather than estimating ECS using an estimate of the PETM forcing change, Hansen calculates what the PETM CO2 level would have had to be to cause warming of 5.6°C, based on his ECS estimate of 4.8°C, assuming a pre-PETM CO2 level of 910 ppm and that non-CO2 greenhouse gases contributed 25% as much forcing as CO2 did. Hansen thereby calculates a PETM CO2 level of 1630 ppm. In fact, the correct figure seems to be approximately1565 ppm; Hansen appears to have gone wrong somewhere in his calculations.
Although Hansen’s 910 ppm pre-PETM CO2 concentration value is closely in line with other estimates, the circa 1600 ppm value for the PETM CO2 concentration implied by his 4.8°C ECS and his other assumptions is much lower than the estimate of 2400 ppm, with a ±1 standard deviation range of 1700–3100 ppm, that Sherwood et al. (2020) reached after assessing the available evidence. It is also near the bottom of the 1400–3150 ppm uncertainty range given in AR6.
Moreover, Sherwood et al. assessed the best estimate GMST rise at the PETM to be slightly lower than did Hansen, at 5.0°C, and the non-CO2 greenhouse gas forcing to be higher, at 40% of CO2 forcing. All these differences contribute to Sherwood et al.’s PETM-based ECS estimate being, at 2.5°C, barely half Hansen’s assumed 4.8°C. Moreover, Lewis (2023), using identical data-variable assumptions as Sherwood et al. (2020) but the AR6 formula for CO2 forcing, which is more accurate at high concentrations than the simple formula Sherwood et al. used, obtains an even lower PETM-based ECS estimate of 2.2°C.
The PETM section of Table 1 in the Appendix gives details of all the data-variable estimates used to form the Sherwood et al. (2020)and Lewis (2023) PETM based ECS estimates, and the Hansen et al. (2023) estimate of CO2 concentration at the PETM. Figure 1 shows what the central estimate of the total change in forcing involved was, as a multiple of the forcing from a doubling of preindustrial CO2 concentration, for all three studies’ LGM and PETM estimates.
Although there are much greater uncertainties involved when estimating ECS from the PETM event than for the LGM – preindustrial Holocene transition, the available PETM evidence is closely consistent with the lower, 2.2°C and 2.8°C, ECS estimates derived from the LGM data-values used respectively by Lewis (2023) and Sherwood et al. (2020), but not with Hansen’s very high 4.8°C estimate of ECS.
It is also clear that Hansen’s claim that today’s human-made greenhouse gas forcing is, at 4.6 W/m2, at least comparable to the PETM forcing (which his assumptions imply was 4.67 W/m2) is strongly at variance with the evidence as assessed by Sherwood et al.17Hansen’s claim here that today’s human-made GHG forcing is 4.6 W/m2 is moreover inconsistent with the statement earlier in his paper that it is 4.13 W/m2 for 2022 (1.03× his value for a doubling of CO2 concentration, close to the AR6-basis ratio), and growing by 0.5 W/m2 per decade. That evidence implies a best estimate of PETM forcing of 1.98× that for a doubling of preindustrial CO2 concentration18Based on the AR6 formula for CO2 forcing (versus 1.17× on Hansen’s assumptions), double or more the latest (2022) AR6-basis estimate, in Forster et al. (2023), of 0.88× for greenhouse gas forcing (1.00× when including that from ozone, a short lived greenhouse gas).
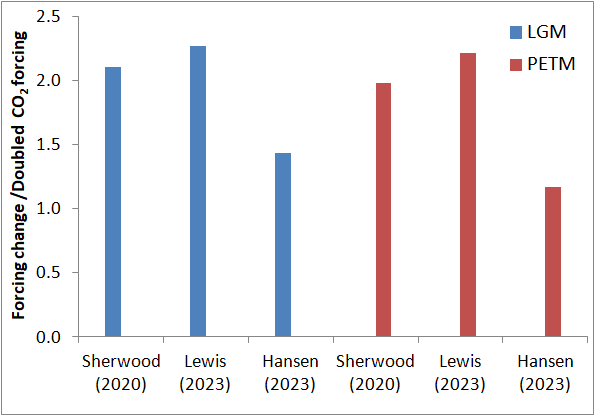
Figure 1.The forcing change between the LGM and preindustrial Holocene, and between before and during the PETM, implicit in each study’s assumptions. The forcing changes are expressed as a multiple of that from a doubling of preindustrial CO2 concentration. The associated ECS estimate equals in each case the corresponding assumed GMST change divided by the forcing change shown, so a higher forcing change implies a lower estimated ECS value.
Conclusions
I do not consider that Hansen’s climate sensitivity estimation properly assesses and fairly reflects all the available relevant evidence. Unfortunately, unlike Sherwood et al.(2020), IPCC AR6 and Lewis (2023), Hansen et. al. (2023) estimates ECS using only paleoclimate proxy-derived evidence, which generally varies considerably according to the proxies involved and to the methods used to interpret them. This opens the door for biased (cherry picked) assessments. For instance, Hansen et. al. do not even mention any studies (e.g. Annan and Hargreaves (2013) and (2022)) that find a much lower LGM – preindustrial warming than their chosen value.
Although I respect Hansen’s ability and considerable scientific contributions, in my view papers he leads are increasingly strongly biased towards overheated projections and dire conclusions.19Moreover, I’m not sure that Hansen is fully up with recent developments in climate science. For instance, when discussing different measures of radiative forcing, a topic on which Hansen wrote a seminal paper in 2005, he wrongly claims that (unlike for the formulae in his equation (3)) AR6 uses the biased-low Fo measure for its long-lived (non-ozone) greenhouse gas effective radiative forcing (ERF) estimates, and on that basis adjusts the AR6 forcing values. The 2013 (AR5) IPCC assessment report did use Fo, but AR6 uses a measure that, like Hansen’s preferred Fs, excludes the effect of surface temperature change. The “political recommendations” with great impact on the society in Hansen et al. (2023) cannot be justified because their foundation is very shaky, as shown here for climate sensitivity and, in relation to warming-in-the-pipeline and ocean heating, in Michael Mann’s critique.
Nicholas Lewis 6 November 2023
Appendix
Table 1. Paleoclimate evidence data-variable best-estimate valuesa used to estimate S and ECS
| Description | Symbol | Sherwood et al 2020 | Lewis 2023 | Hansen et al 202320Hansen estimates ECS from changes in greenhouse gases (GHG) between the LGM and the mid-Holocene. The GMST estimate he uses is the same for that period as for the immediately preindustrial period (circa 1750). However, changes in non-GHG forcing agents are more uncertain for the mid-Holocene than for the preindustrial period (which most forcing change estimates relate to). In particular, aerosols and land surface albedo could have been significantly affected by deforestation starting well before the preindustrial period, a possibility mentioned in Hansen’s paper. This makes ECS estimates based on LGM to mid-Holocene changes more uncertain than those based on LGM to preindustrial changes. | Comment re Hansen |
| ERF from doubled CO2 (W/m2) | F2×CO2 | 4.00 | 3.93 | 4.00 | |
| LGM | |||||
| Change in GMST (°C) | ΔT | −5.0 | −4.5 | −7.0 | |
| Changes in forcing, as ERF (W/m2) | |||||
| CO2 | −2.28 | −2.24 | See GHG | ||
| Methane (CH4) | −0.57 | −0.57 | See GHG | ||
| Nitrous oxide (N2O) | −0.28 | −0.28 | See GHG | ||
| Total greenhouse gas (GHG) | −3.13 | −3.09 | −2.25 | ||
| Land ice and sea level | −3.20 | −3.72 | |||
| Vegetation | −1.10 | −1.10 | |||
| Dust (aerosol) | −1.00 | −1.00 | 0 | Excluded | |
| Forcing excluding that from GHG | ΔFexCO2 | −5.30 | −5.82 | −3.5 | |
| Change in total forcing | ΔF | −8.43 | − 8.92 | -5.75 | |
| Dependence of feedback on ΔT (W/m2/°C2) | α | 0.10 | 0.10 | 0 | |
| Resulting estimate of ECS (°C) | ECS | 2.79 | 2.24 | 4.87 | |
| PETM | |||||
| Change in GMST (°C) | ΔT | 5.0 | 5.0 | 5.6 | |
| Fractional change in CO2 concentration | ΔCO2 | 1.667 | 1.667 | 0.72 | Implied |
| CO2 ERF relative to with log(concentration) | fCO2nonLog | Ignored | 1.117 | 1.19 | See note b |
| CH4 forcing as a fraction of that from CO2 | fCH4 | 0.40 | 0.40 | 0.25 | |
| Change in total forcing | ΔF | 7.93 | 8.70 | 5.75 | |
| Estimate of ECS (°C) | ECS | 2.52 | 2.26 | 4.8 | Assumed |
Notes:
a Data-values for Sherwood et al.(2020) and Lewis (2023) are medians that have been extracted from Table 3 of Lewis (2023), the notes to which are incorporated here by reference. See those papers for details of the sources used to derive their respective data-variable estimates.
b Using the Hansen et al. (2023) Table 1 formula for CO2 forcing
- 1ECS is defined as the global mean surface warming for a doubled CO2 concentration after so-called fast-feedbacks have been fully activated and the ocean has reached equilibrium (which it approaches within a thousand or so years). Earth system sensitivity (ESS), which also includes very slow feedbacks such as those from changes in ice sheets, represents global warming arising over much longer timescales. ESS is relevant when studying paleoclimate changes, but not when projecting changes over the next few centuries.
- 2E.g., in Hansen et al (2013) Climate sensitivity, sea level and atmospheric carbon dioxide. Phil Trans Roy Soc A. https://doi.org/10.1098/rsta.2012.0294
- 3Some of the latest generation (CMIP6) global climate models do have ECS values above 5°C, but they are generally regarded by climate scientists as being too sensitive.
- 4Actually two slightly different versions, iCESM1.2 and iCESM1.3, of the same underlying CESM1 GCM.
- 5Supporting my critique of their reanalysis, I showed that its co-located estimates were uncorrelated with values from the cave speleothem proxies it used. Moreover, unlike proxy-based reconstructions (e.g., Caufman et al. (2020)), which show early Holocene GMST 5,000–9,000 years ago being circa 0.5°C higher than late preindustrial (1750) GMST, their reanalysis showed that period as being significant cooler than late preindustrial GMST.
- 6The final, published version of Osman et al. was slightly different, with no mention of the change in the peer review files, and cooled marginally more near and at the LGM.
- 7Using a more recent model generation than the older ones Osman et al. used to derive the ratio used to convert their ex-polar sea surface temperature (SST) proxy-based estimates to GMST.
- 8No explanation is available of why, disconcertingly, this differs from the 5.9°C in their submitted manuscript.
- 9The Osman et al. cooling estimate may be greater because they use only a subset of Tierney et al.’s proxies, and have no proxy coverage at all in the central Pacific ocean.
- 10While Seltzer et al. validated their model on modern proxy data, it may not be valid for conditions at the LGM, when factors such as vegetation cover, rainfall patterns, and seasonal temperature and precipitation cycles were different. An additional concern is that Seltzer use two alternative methods to select LGM samples, which give noticeably different LGM cooling estimates (5.8°C and 4.8°C). They prefer the method giving the higher estimate, however it will tend to pick out particularly cold temperatures from datasets, and so may overestimate LGM cooling. Interestingly, the average of the Annan et al. LGM reconstruction’s cooling estimates for grid cells co-located with the 15 proxies that Seltzer et al. use is close to the 4.8°C estimate that Seltzer et al. obtain using their second method.
- 11Thus, excluding two particularly cool, low assessed uncertainty proxy estimates would reduce the estimate by 0.4°C.
- 12Lewis (2023) was submitted earlier than was Annan et al (2022).
- 13Lewis, N., 2023. Objectively combining climate sensitivity evidence. Climate Dynamics, 60(9), pp.3139-3165.
- 14Hansen’s estimate of the greenhouse gas forcing change, relative to that from a doubling of CO2 concentration, is 27% lower than the value assessed by Sherwood et al. and also adopted in Lewis (2023). Moreover, Hansen ignores the significant dust aerosol forcing change assessed by Sherwood et al., likewise also adopted in Lewis (2023).
- 15In the formula for estimating ECS, the change in forcing (ΔF) forms the denominator: ECS = F2×CO2 × ΔT/ΔF, where ΔT is the GMST change in equilibrium and F2×CO2 is the forcing from a doubling of atmospheric CO2 concentration. All forcing changes given in this article are for effective radiative forcing (ERF), the principal forcing metric used in AR6.
- 16Sherwood et al. (2020) and Lewis (2023) focused on a climate sensitivity measure, S, an easier to estimate approximation to ECS that is normally derived for global climate models instead of ECS. They accordingly converted their underlying paleoclimate ECS estimates into estimates of S.
- 17Hansen’s claim here that today’s human-made GHG forcing is 4.6 W/m2 is moreover inconsistent with the statement earlier in his paper that it is 4.13 W/m2 for 2022 (1.03× his value for a doubling of CO2 concentration, close to the AR6-basis ratio), and growing by 0.5 W/m2 per decade.
- 18Based on the AR6 formula for CO2 forcing
- 19Moreover, I’m not sure that Hansen is fully up with recent developments in climate science. For instance, when discussing different measures of radiative forcing, a topic on which Hansen wrote a seminal paper in 2005, he wrongly claims that (unlike for the formulae in his equation (3)) AR6 uses the biased-low Fo measure for its long-lived (non-ozone) greenhouse gas effective radiative forcing (ERF) estimates, and on that basis adjusts the AR6 forcing values. The 2013 (AR5) IPCC assessment report did use Fo, but AR6 uses a measure that, like Hansen’s preferred Fs, excludes the effect of surface temperature change.
- 20Hansen estimates ECS from changes in greenhouse gases (GHG) between the LGM and the mid-Holocene. The GMST estimate he uses is the same for that period as for the immediately preindustrial period (circa 1750). However, changes in non-GHG forcing agents are more uncertain for the mid-Holocene than for the preindustrial period (which most forcing change estimates relate to). In particular, aerosols and land surface albedo could have been significantly affected by deforestation starting well before the preindustrial period, a possibility mentioned in Hansen’s paper. This makes ECS estimates based on LGM to mid-Holocene changes more uncertain than those based on LGM to preindustrial changes.
